

The first row, r 1 = (1, 1, 3), corresponds to the first equation, 1 x + 1 y = 3, and the second row, r 2 = (3, −2, 4), corresponds to the second equation, 3 x − 2 y = 4. This is called the augmented matrix, and each row corresponds to an equation in the given system. Next, the coefficient matrix is augmented by writing the constants that appear on the right‐hand sides of the equations as an additional column: This is called the coefficient matrix of the system. The first step is to write the coefficients of the unknowns in a matrix: The previous example will be redone using matrices. This method reduces the effort in finding the solutions by eliminating the need to explicitly write the variables at each step. Gaussian elimination is usually carried out using matrices. (Back‐substitution of y = 1 into the original second equation, 3 x − 2 y = 4, would also yeild x = 2.) The solution of this system is therefore ( x, y) = (2, 1), as noted in Example 1. Back‐substitution of y = 1 into the original first equation, x + y = 3, yields x = 2. This final equation, −5 y = −5, immediately implies y = 1. Multiplying the first equation by −3 and adding the result to the second equation eliminates the variable x: This method, characterized by step‐by‐step elimination of the variables, is called Gaussian elimination. Once this final variable is determined, its value is substituted back into the other equations in order to evaluate the remaining unknowns. The fundamental idea is to add multiples of one equation to the others in order to eliminate a variable and to continue this process until only one variable is left. I could have written them closer to their equal sign.The purpose of this article is to describe how the solutions to a linear system are actually found. Just so you can see the corresponding, but obviously, And just like that, we'veĪctually solved our system of three equations with
Augmented matrix plus#
And then finally, we haveĠx plus 0y plus 1z is equal to minus 1. So what do we get? We get x plus 0y plusĠz is equal to 5. And actually, I haveĪugmented matrix world and kind of put back our Successive row is to the right of the pivot entry before it. My pivot entries are the onlyĮntries in their columns. Minus 2, or minus 3 plus 2, which is equal to minus 1. So what is that equal to? This is equal to minus 3 minus I can do is I'll replace it with the second row minusĢ times the first row. My first row equal to my first row plus my last row,īecause if these two add up, they're going toīe equal to 0. So then this becomes a plus 1Īnd that becomes a minus 1. Let me just multiply this equation by minus 1. This has to be a positiveġ in order to get there. Sure I didn't make a careless mistake there. 3 minus 2 times 2, that'sģ minus 4, or minus 1. 2 minus 2 times 1, that'sĢ minus 2, that's 0. With the third row minus 2 times the second row.

Now, let me get rid of thisĮntry right here. I always want to make sure Iĭon't make a careless mistake. That's equal to 3 plus 3, so that's equal to 6. So where was I? I'm replacing the first row with I can do is I can replace the first row with the first row Then I have a minus 3, the augmented part of it. Now, I need to target thisĮntry and that entry. Which is what I want for reduced row echelon form. Guy with this equation minus that equation. Second row minus the first row instead of the first row 1 minus 2 is- actually, aīetter thing to do, because I eventually want this to be 1Īnyway, let me replace this row with this row, with the Just replace the second row with the first row minus So it will just be a 1, a 1, aġ, and then my dividing line, and then I have a 3.
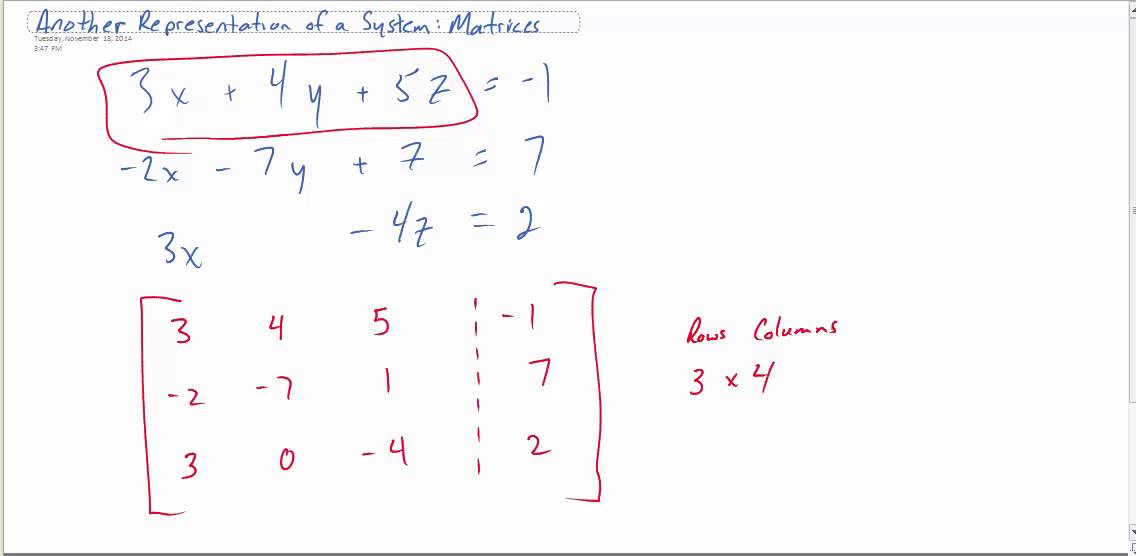
So the first thing, I haveĪ leading 1 here that's a pivot entry. Now, I want to get thisĪugmented matrix into reduced row echelon form. Coefficients of the z termsģ, 0, and minus 2. Coefficients of the y termsĪre 1, 2, and 3. So what's the augmented matrixįor this system of equations? Three unknowns with Going to solve it using an augmented matrix, and I'm going Linear equations, so let's solve this one. As much practice as possible solving systems of


 0 kommentar(er)
0 kommentar(er)
